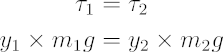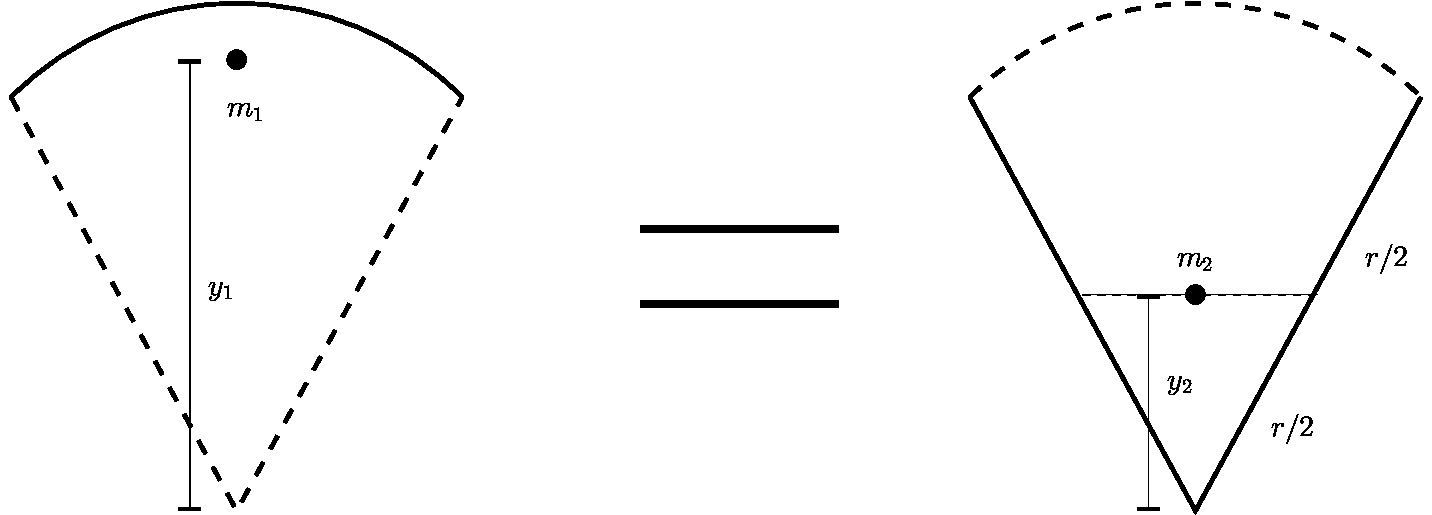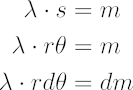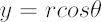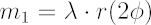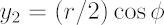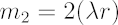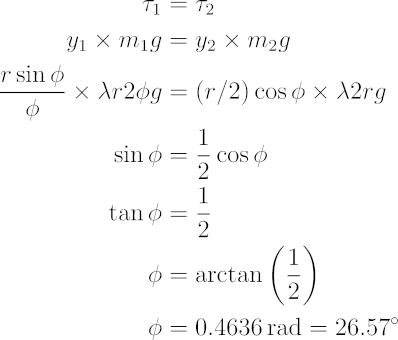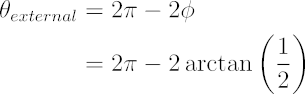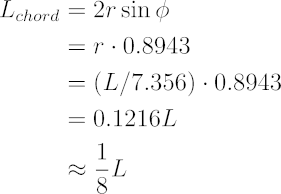The Sakai Top
I've come across the rather fascinating Sakai Top, a top made from a single wire. The top was described in 1986 by Takao Sakai, a professor of mechanical engineering at the Fukushima Technology Centre of Kohriyama-City [1]. The top is also known as the paperclip top, since it is often made with paperclips. The physics behind the top is often "left up to the reader as an exercise."
The Physics Exercise of the Top
The Sakai Top is constructed such that the the spokes are balanced by the missing arc in the top [2]. In other words, the total gravitational torque on the spokes is equal to the gravitational torque on the material that would have filled the missing arc. Let the subscript 1 denote descriptions for the missing arc, and subscript 2 denote descriptions for the spokes.
Here, we see we need four quantities. y1 is the center of mass of the "missing" arc, and y2 is the center of mass of the spokes. y1 and y2 are distances away from the center of the top. Note that we assume that the top is symmetric about the bisector of the angle formed by the spokes, so both centers of mass lie on this bisector. m1 is the mass of the arc, while m2 is the total mass of the spokes. Both masses are modeled as point masses. Finally, g is the gravitational acceleration.
Description of the Missing Arc
Let's look at arc's center of mass, y1. The center of mass is the weighted average of positions along the angle bisector using the masses at each position as the weights.
First, dm is a small change in mass, which proportional to a very small arc s it belongs to. λ is the mass per length of the wire. Arc length s is described by the top's radius r and a very small angle θ.
Second, the position along the bisector y can be described in terms of an angle θ and radius r with trigonometry.
With these two descriptions, we can solve for the center of mass of the arc. Recognizing the symmetry along the bisector, we can integrate from 0 to ϕ, which we'll define as half of the angle formed by the spokes. The angle is referenced from the bisector.
We can describe the mass of the arc m1 with its mass density and length, as done previously. Note that ϕ is half of the angle of the arc, so we need 2ϕ to describe the full arc.
With y1 and m1, we can describe the torque due to the "missing" arc.
Description of the Two Spokes
Let's look at spokes' center of mass, y2. We take a shortcut around integrating for y2 by seeing that half of the mass lies below half of the radius, while the other half of the mass lies above half of the radius. The center of mass lies on this midpoint projected onto the bisector of the spokes' angle.
The mass of the spokes, m2, is the product of the mass density and the total length of the two spokes.
Equating the Torques
The problem is now fully constrained, and we can find a specific dimension that makes balance possible.
ϕ is half of the angle between the spokes, and it must be 26.57° for the top to balance. The full arc angle is twice of ϕ, which is 53.13°.
An Engineering Description
In terms of practicality, the angle ϕ is not very useful when it comes to actually making a top. Who actually has a protractor on hand? Since you start building the top with a certain length of material and probably have a ruler somewhere, it makes sense to describe the balance condition as a length instead of an angle.
From a length of material, we'll form the top's tip, handle, and the spokes with the not-missing arc. Let's describe the length taken up by the spokes and the existing arc with L. The tip and the handle are not constrained by the balance condition, so we don't need to focus too much those lengths.
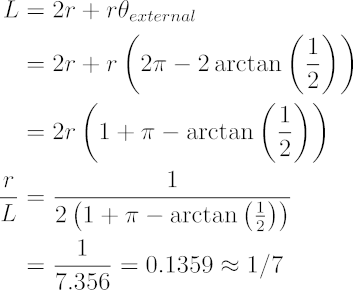
The length L, which affects the balance of the top, consists of the two spokes and the existing arc.
The existing arc exists over the external angle, θ_external, formed by the two spokes. We can describe the external angle in terms of the internal angle 2ϕ that we previously found.
Now, we find the balance condition in terms of the length L of material we are using.
Using this radius-to-length ratio, we can replace a protractor with a ruler and a calculator (or multiplication skills) to find the radius from a given length of material. If you are particularly keen on geometry, you may skip multiplication altogether and use the approximated 1/7 with methods of dividing a line segment into n equal parts (this does require a compass, though). The ratio 1/7 deviates only 5.12% from the actual value.
Measurements for Verification
The process of manually bending a wire into a top is difficult to do right. Fortunately, just a couple of measurements can show if the top is correct.
The first measurement is to make sure the top is round with the correct radius. Measure the radii around the whole top to make sure it's right.
The second measurement is done to verify the angle. Since the angle is difficult to measure without a protractor, we can use the length of the chord produced by the two spokes to verify the angle. We can also represent the chord length in terms of the radius, which is proportional to the original length L.
The approximated chord-to-length ratio 1/8 is off by only 2.80%.
In Summary
An Example Build
Here lies a common paperclip. I'll be using a pair of pliers and a caliper.
I begin by uncurling the paperclip. It seems that the paperclip gives about 10 cm of material.
I make the tip and handle of the top. In general, keeping the handle and tip short will give you more material to form the body of the top, which will increase its rotational moment of inertia and make the top spin for a longer time. After bending the handle and tip, a length L = 85.65 mm is left.
Using our derived ratio, I find the radius r = 11.64 mm. I make the bends for the spokes to be the length of the radius.
After making the spokes, all that's left is the main arc. I make many very small bends along the wire with my pliers (patience is the key). The chord of the completed top according to the previous calculations should be 10.41 mm.
The result is a very well balanced top.